Resistance to the low-density regions: the Hodges-Lehmann location estimator based on the Harrell-Davis quantile estimator

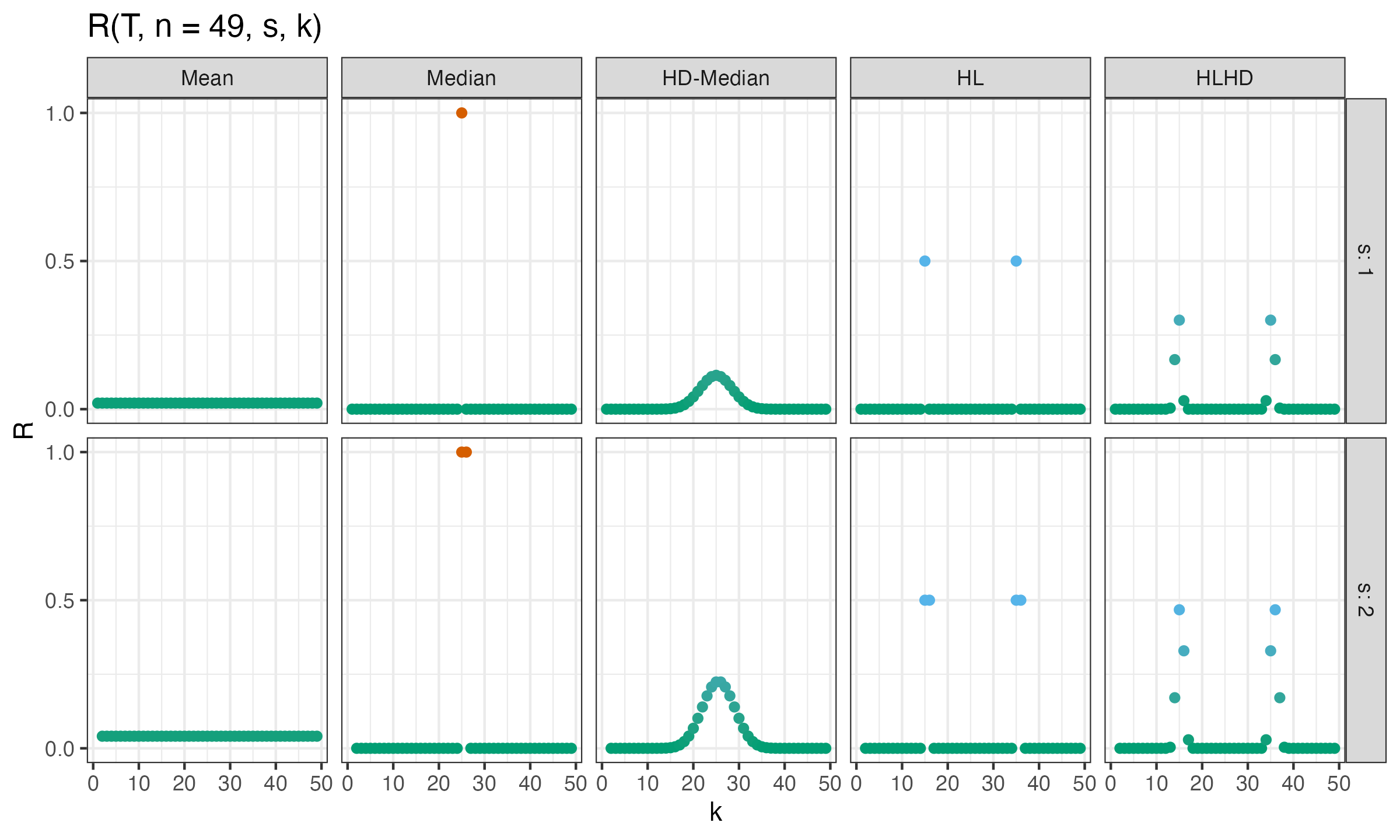
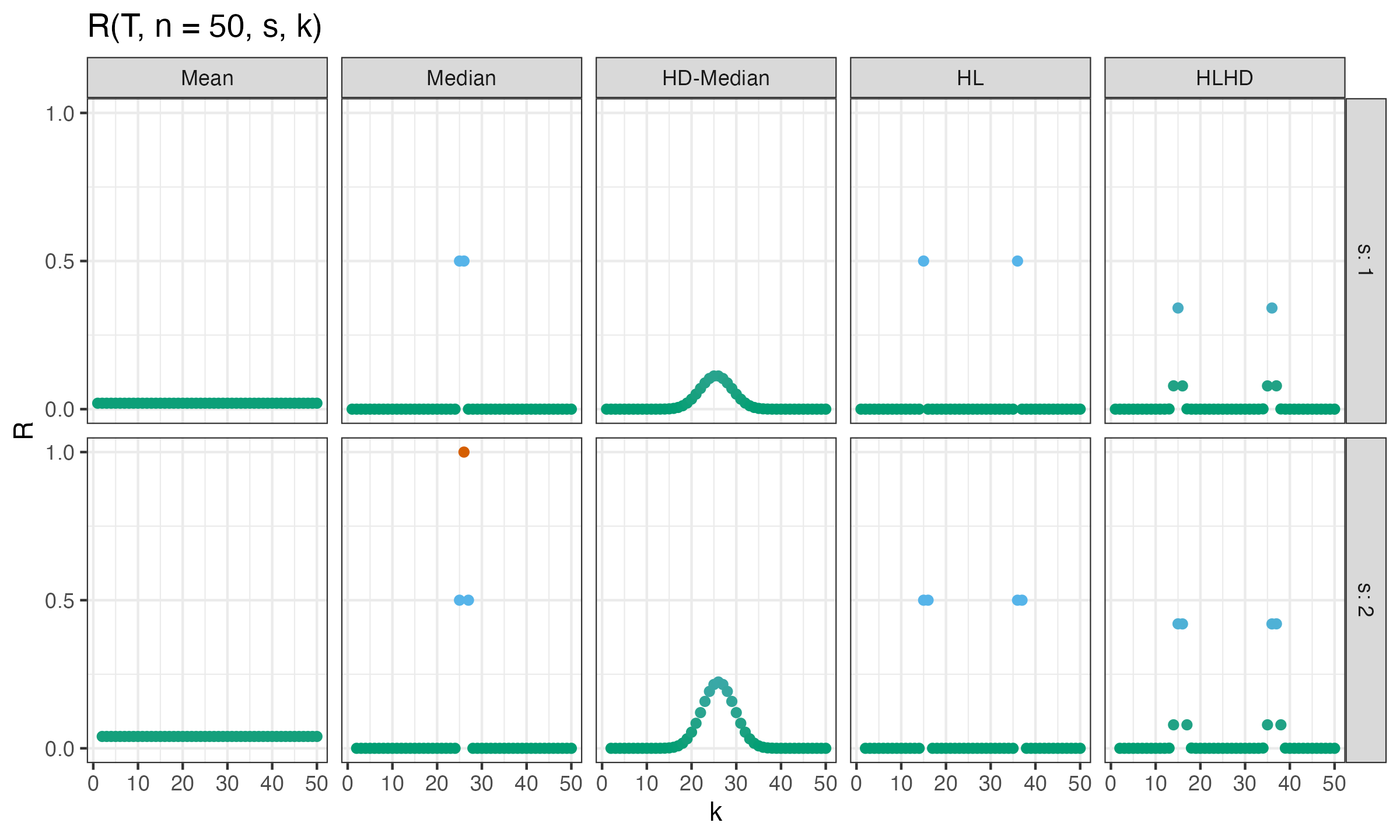
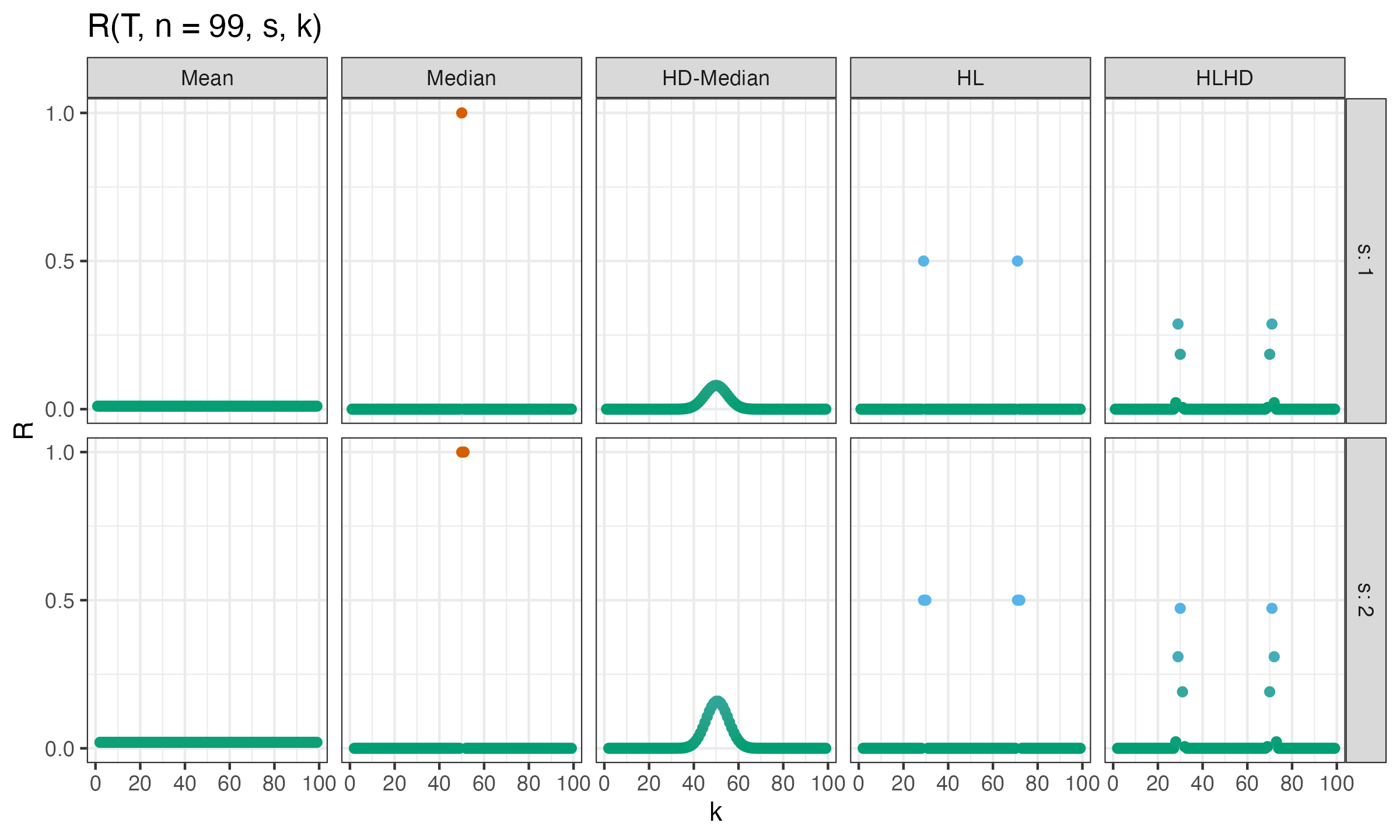
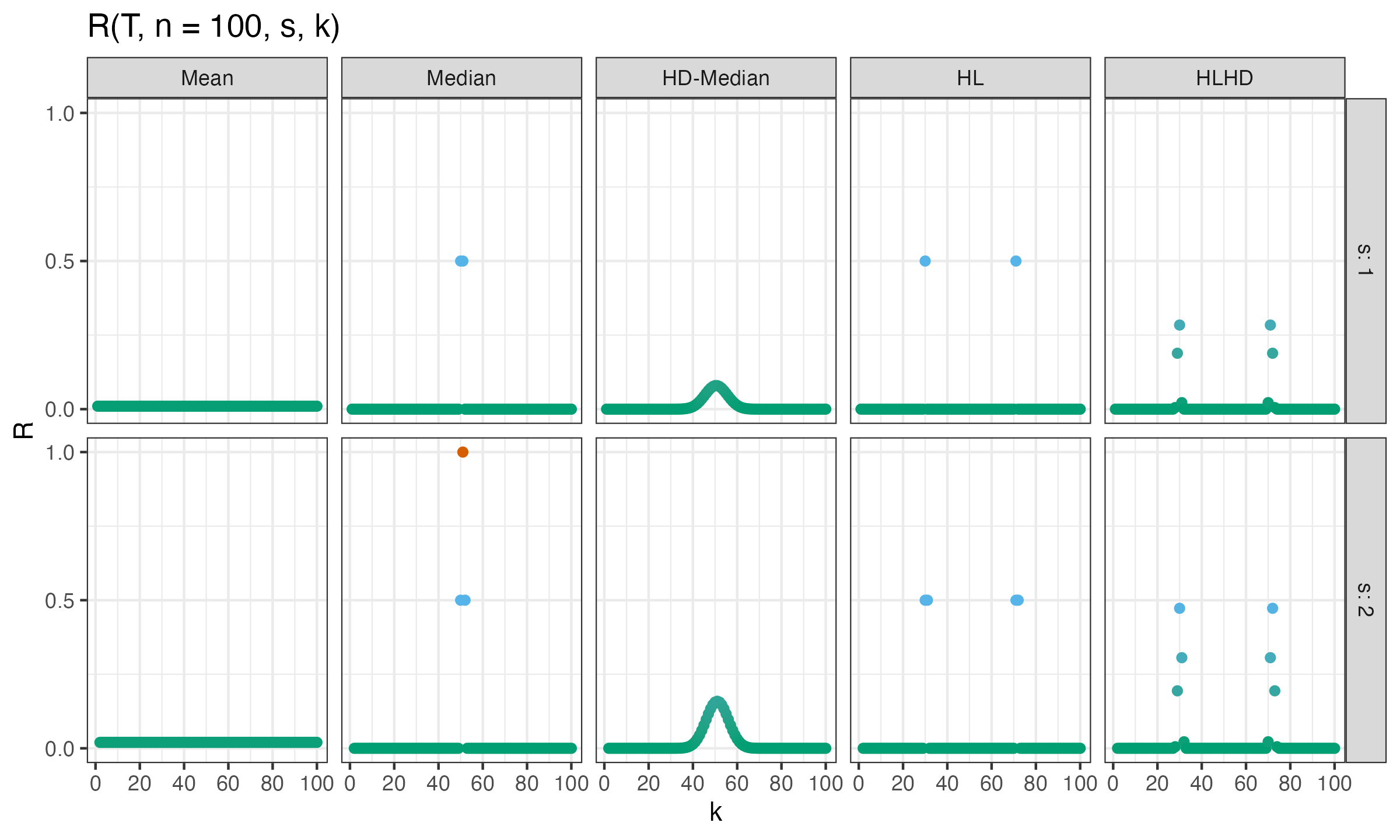
Previously, I have discussed the topic of the resistance to the low-density regions of various estimators including the Hodges-Lehmann location estimator ($\operatorname{HL}$). In general, $\operatorname{HL}$ is a great estimator with great statistical efficiency and a decent breakdown point. Unfortunately, it has low resistance to the low-density regions around $29^\textrm{th}$ and $71^\textrm{th}$ percentiles, which may cause troubles in the case of multimodal distributions. I am trying to find a modification of $\operatorname{HL}$ that performs almost the same as the original $\operatorname{HL}$, but has increased resistance. One of the ideas I had was using the Harrell-Davis quantile estimator instead of the sample median to evaluate $\operatorname{HL}$. Regrettably, this idea did not turn out to be successful: such an estimator has a resistance function similar to the original $\operatorname{HL}$. I believe that it is important to share negative results, and therefore this post contains a bunch of plots, which illustrate results of relevant numerical simulations.

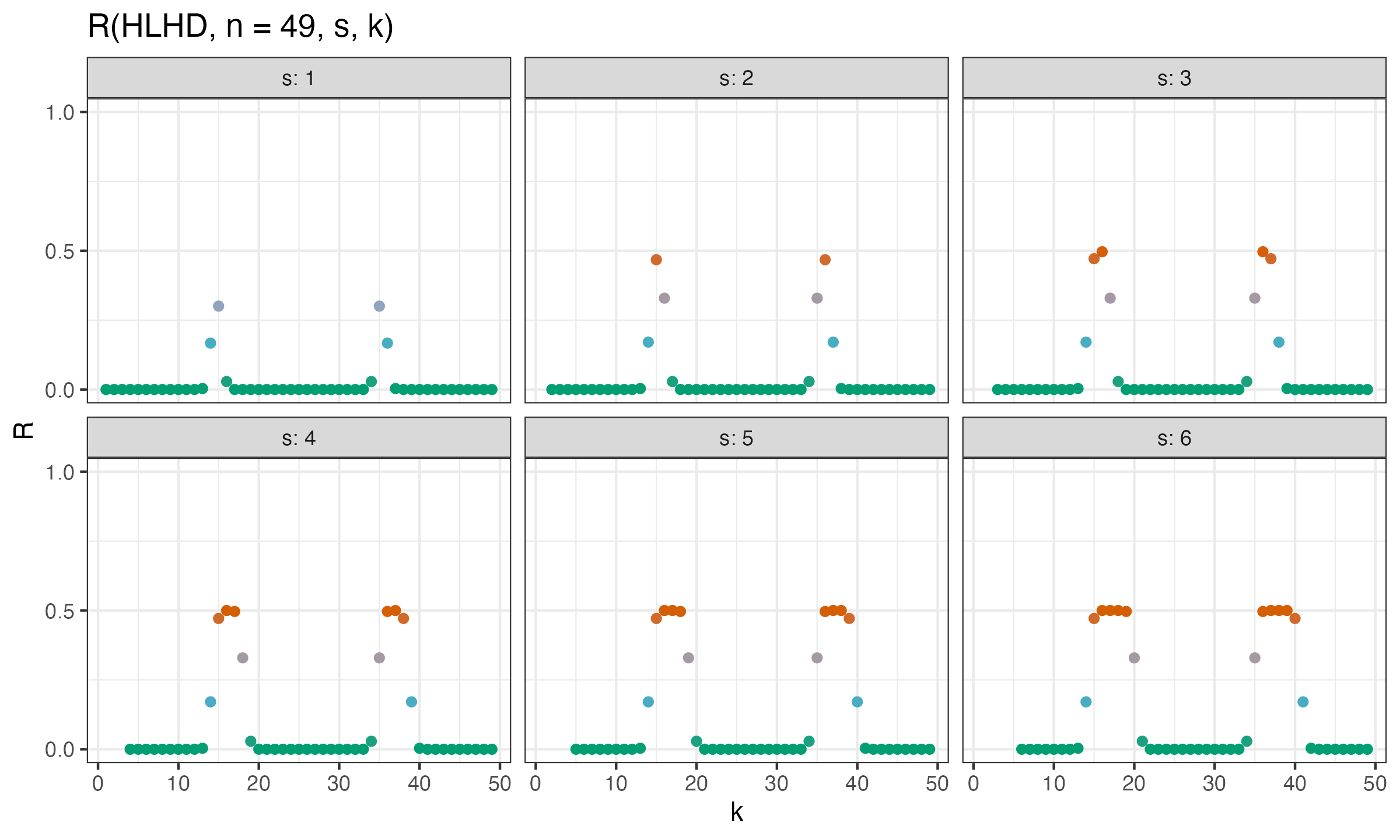
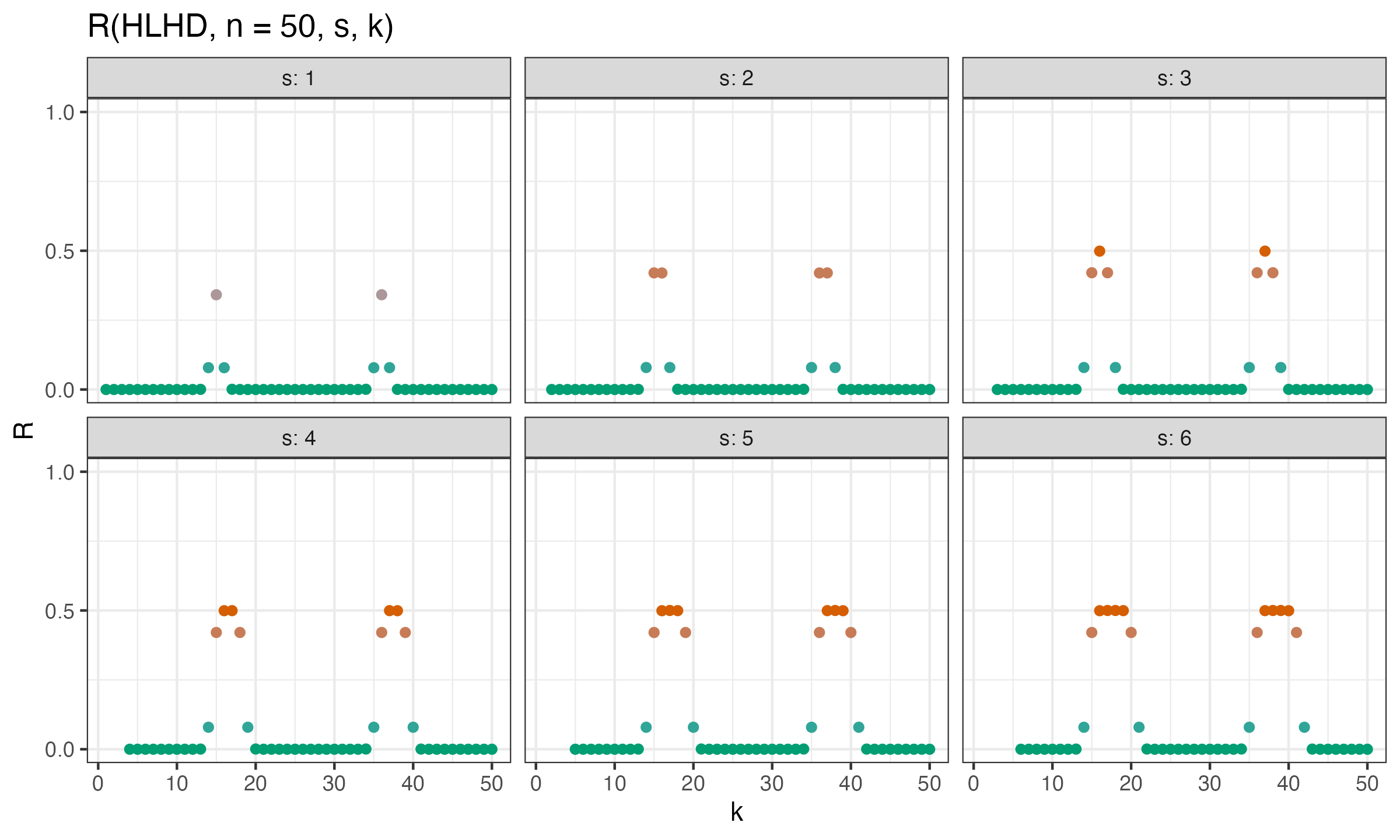
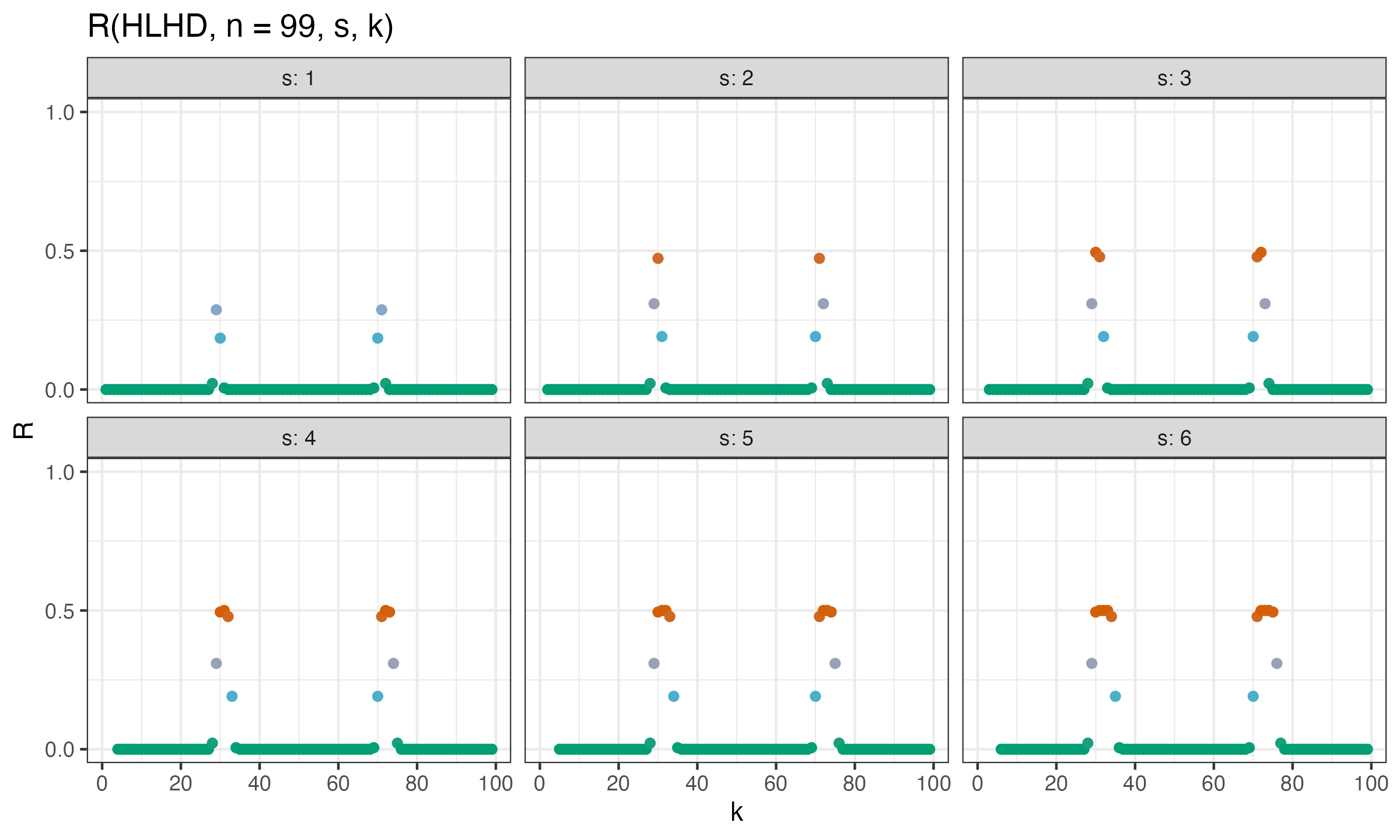
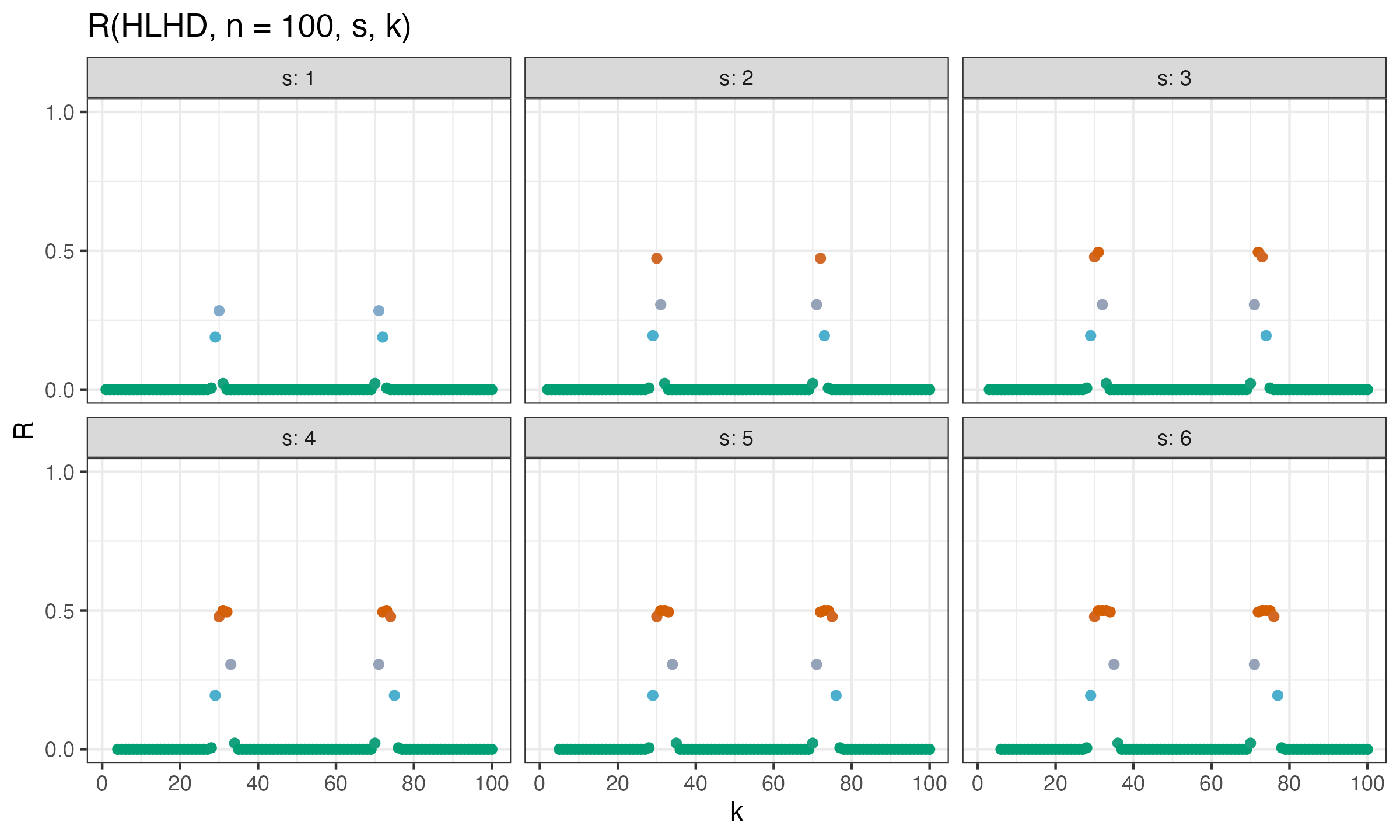
In this post, I extend research from the original post about $\operatorname{HL}$ resistance by adding a new estimator that we denote as $\operatorname{HLHD}$. The simulation setup is fully reused from the previous post. Here are the updated results: